Calculating Energy and Work
Equations you need to know for IGCSE Physics:
GPE = mgh
GPE = gravitational potential energy (J)
m = mass (kg)
g = gravitational acceleration (10 m/s2)
h = change in height (m)
KE = (1/2)mv2
KE = kinetic energy (J)
m = mass (kg)
v = velocity (m/s)
W = Fd
W = work done (J)
F = force (N)
d = distance moved (m)
P = W / t
P = power (W)
W = work done or energy transferred (J)
t = time (s)
Example 1
A large rock of mass 50kg is lifted 80m to remove it from the mine.
(a) Calculate the gain in gravitational potential energy when the rock is lifted 80m.
GPE = 50 x 10 x 80 = 4.0 x 104 J
(b) State the work done in lifting the rock.
Work done against gravity = change in gravitational potential energy = 4.0 x 104 J
Example 2
A truck travels up a hill at constant speed. The diagram shows the dimensions of the hill.
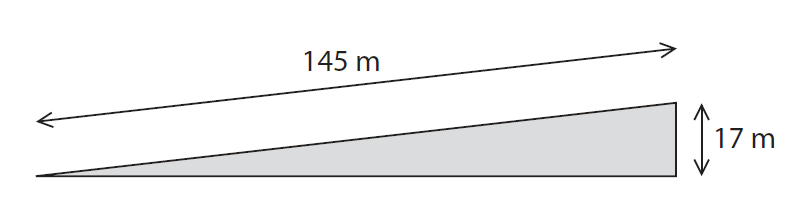
(a) The weight of the truck is 180kN. Calculate the work done against gravity by the track in moving to the top of the hill.
Work done against gravity = gain in gravitational potential energy = 180 x 1000 x 17 = 3.1 x 109 J
(b) The truck takes 8.0s to travel up the hill. Calculate the useful power of the truck.
power = 3.1 x 109 / 8.0 = 3.8 x 108 W
Example 3
A raindrop of mass 0.000035kg falls 1200m above the ground.
(a) Calculate the change in gravitational potential energy.
GPE = 0.000035 x 10 x 1200 = 0.42 J
(b) State the kinetic energy of the raindrop as it hits the ground. Assume no energy losses.
Without any energy loss, all of gravitational potential energy is transferred to kinetic energy. Therefore, kinetic energy is equal to 0.42 J.
(c) Show that the speed of the raindrop as it hits the ground would be about 150m/s. Assume no energy losses.
KE = (1/2)mv2
v2 = (2 x KE) / m = 2 x 0.42 / 0.000035 = 24000
v ? 150 m/s
(d) Explain why the actual speed of the raindrop as it hits the ground is much less than 150m/s.
- There is air resistance acting on the raindrop as it falls.
- Energy is lost surroundings, so not all of the initial gravitational potential energy is transferred as kinetic energy.
- Since the air resistance acts upwards, the resultant downwards force on the raindrop is less.
- The raindrop reaches terminal velocity when the magnitude of gravitational force and air resistance becomes equal.
Example 4
(a)A machine lifting a load uses an average (mean) power of 1.9 MW to do 67 MJ of work. Calculate the time needed to do this work.
P = W / t
t = W / P = (67 x 106) / (1.9 x 106) = 35 s
(b) State the effect of using a lower average power to do this work.
Using a lower average power to do the same work will take more time to do the work.
Example 5
An underground train enters a station.
(a) The mass of the train and its passengers is 250,000 kg. The total kinetic energy is 18 MJ.
(i) Calculate the velocity of the train as it enters the station.
KE = (1/2)mv2
v2 = (2 x KE) / m = 2 x 18 x 106 / 250000 = 144
v = 12 m/s
(ii) The driver applies the brakes to stop the train. State what happens to the kinetic energy of the train.
Energy is lost to the surrounding as heat and sound, so kinetic energy of the train decreases.
(b) The diagram shows a section through the station.
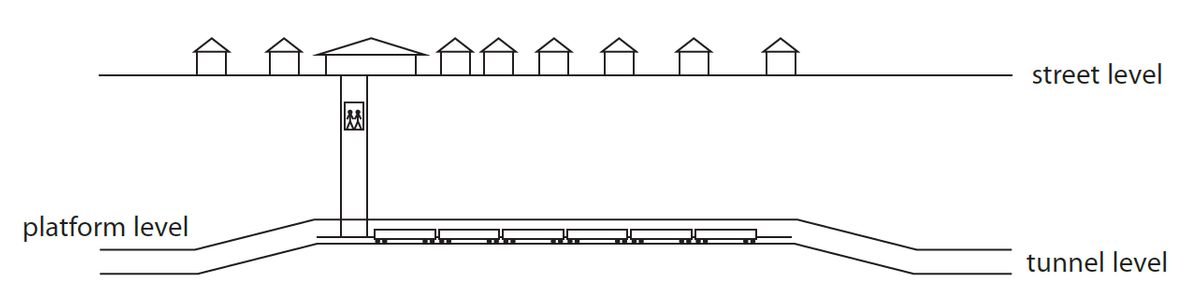
(i) The passengers who use the station are carried from platform level to street level in a lift. Explain why these passengers gain gravitational potential energy in the lift, even when they are below ground.
- GPE = mgh; when using this equation, h refers to the change in height.
- Passengers have moved to a higher point when they are carried from platform level to street level.
- Since gravitational force still acts underground, work is done on the passengers against gravity to lift them to a higher point.
(ii) The tunnel is designed so that the trains go up a slope as they enter the station and go down a slope as they leave. The driver uses brakes to stop the train in the station and a motor to make the train move away. Explain how the sloping parts of the tunnel affect the amount of work that needs to be done on the train by the brakes and by the motor.
When entering the station:
- Kinetic energy of the train is reduced as it is transferred to gravitational potential energy as the train goes up the slope.
- Less braking force is needed to stop the train.
- Less work is done by the brakes.
- The brakes can be applied for a shorter period of time.
When leaving the station:
- Kinetic energy of the train is increased as gravitational potential energy of the train is transferred as kinetic energy as the train goes down the slope.
- Less force is needed to accelerate the train.
- Less work is done by the motor.
- The train accelerates more quickly.
|